Project Description
Matlab Professional Assignment help sample
Multiple?Antennas?and?Space-time?Communications?
Consider a 2??2 MIMO system with channel gain matrix ?? given by
?? = ?
0.3 0.8
0.4 1.5
?
Assume that ?? is known at both transmitter and receiver and that there is a total transmit
power of ?? = 12 ???? across the two transmit antennas, AWGN with ??0 = 10-9 ??/???? at
each receive antenna, and bandwidth ?? = 1??????.
Write a MATLAB script that can,
a) Find the SVD for ??.
(20 marks)
b) Find the capacity of this channel.
(20 marks)
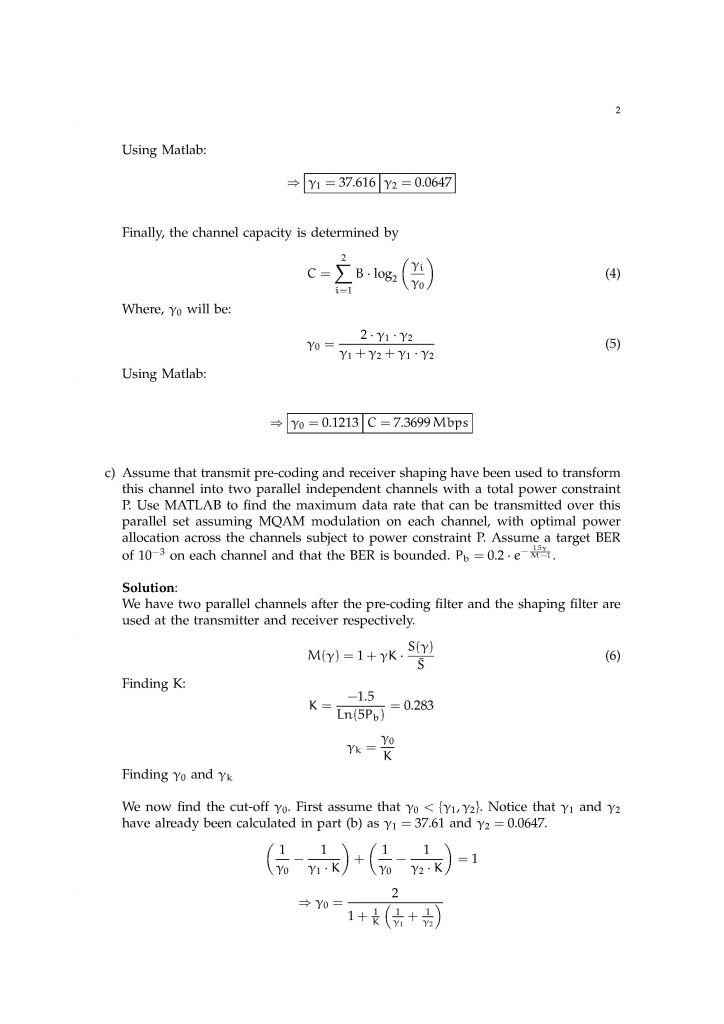
c) Assume that transmit precoding and receiver shaping have been used to transform
this channel into two parallel independent channels with a total power constraint ??.
Use MATLAB to find the maximum data rate that can be transmitted over this
parallel set assuming MQAM modulation on each channel, with optimal power
allocation across the channels subject to power constraint ??. Assume a target BER
of 10-3 on each channel and that the BER is bounded ???? = 0.2??- 1.5??
?? -1.
(20 marks)
d) Suppose now that the antennas at the transmitter and receiver are all used for
diversity (with optimal weighting at the transmitter and receiver) to maximize the SNR of
the combiner output. Using MATLAB, find the SNR of the combiner output as well as the
BER of a BPSK modulated signal transmitted over this diversity system. Compare the data
rate and BER of this BPSK signalling with diversity (assuming ?? = 1/????) to the rate
and BER from part c.
(20 marks)
e) Discuss the diversity-multiplexing trade-offs between the systems in parts c and d.
(20 marks)
Submission Requirements
1. This is an individual assignment; please ensure the submitted work is your own as each
assignment will be tested for academic integrity.
2. The assignment report is to be submitted electronically through QUT Blackboard (due 31st
May 2016).
3. The written report should provide responses to all required tasks, as well as the MATLAB
scripts used to produce your results.
4. The electronic files submitted must be in a pdf file format. This ensures that your document
formatting is preserved.
5. Do not zip or archive your submissions.
6. It is permissible to submit your assignment to Blackboard multiple times.
7. However, only the latest submission will be marked.
8. It is the student’s responsibility to check that files submitted to Blackboard have not been
corrupted in the process. All uploaded files can be reviewed post submission.
%%%%%%%%%%%%%%%%%%%%%%%%%%%
%ENN522
%Assignment
%%%%%%%%%%%%%%%%%%%%%%%%%%%
%%%%%%%%%%%% Variables declaration %%%%%%%%%%%%
clear all
close all
clc
H = [0.3 0.8; 0.4 1.5]; % Matrix H
B = 1e6; % Bandwidth
P = 12e-3; % Transmit power
No = 1e-9; % AWGN Channel
Pb = 1e-3; %Bit error probability ~ BER
%%%%%%%%%%%% Question a %%%%%%%%%%%%
[U,S,V] = svd(H); % Computing (SVD)
Hcmp = U*S*V’; % Checking the relation H = U*S*V’
%%%%%%%%%%%% Qestion b %%%%%%%%%%%%%
gamma1 = (S(1)^2*P)/(No*B); % Gamma1 coefficent
gamma2 = (S(4)^2*P)/(No*B); % Gamma1 coefficent
gamma0 = (2*gamma1*gamma2)/(gamma1 + gamma2 + gamma1*gamma2); %Gamma0 coefficent
C = B * log2(gamma1/gamma0) + B * log2(gamma2/gamma0); % Channel capacity
%%%%%%%%%%%% Qestion c %%%%%%%%%%%%%
K = -1.5/(log(5*Pb)); % M-1 value
gamma0k = 2/(1 + (1/K)*(1/gamma1 + 1/gamma2)); %gamma0k value
gammak = gamma0/K;
R = B*(log2(gamma1/gammak) + log2(gamma2/gammak)); %Bit rate R
%%%%%%%%%%%% Qestion d %%%%%%%%%%%%%
lambdamax = 0.7592; % Max Wishart Matrix value
Tb = 1e-6; %Bit time
rho = P/(No*B); %Adimenssional factor
gamma_s = lambdamax*rho; %Gamma for QPSK scheme
PbBPSK = qfunc(sqrt(2*gamma_s)); %Probability bit error Pb
R_QPSK = 1/Tb; %Bit Rate R
Report
Project Details
- Date December 6, 2016
- Tags Matlab, Programming