Bonds and stock Valuation Assignment Help
Long term securities are those securities which are generally held for the period of more than one year. There is no static rule about the period of long term securities. But normally long term securities are held as little as one year, and as long as 30 years. Some common examples of long term securities are stocks, bonds etc. For sound concept, and well understanding valuation of long term securities, it is necessary we must understand some important terminologies which are commonly use. Therefore, here under we elaborate each terminology separately.
Market value:
Market value of any security means, the market price at which an asset trade. In simple words, Market value is the current price at which a security can buy or sell. Market price is not stagnant, and change according demand and supply conditions.
Liquidation value:
It is the amount of money that could be realized. If an asset or sometime group of assets sell separately from its operation. In other words, it is estimated value which will receive, if the specific asset would be sold out. Additionally, the liquidation value calculated for financial instrument normally at the time of bankruptcy.
Going concern value:
The amount a firm could be sold for as a continuing business operation. When going concern value calculated, it is assume that business continue its operations for the foreseeable future. In short, the ability of a business which will carry on producing positive cash flows in the forthcoming.
Book value:
Book value of a firm calculated through total assets minus total liabilities and preferred stock as listed on the balance sheet. Therefore, asset point of view book value determined subtracted the amount of depreciation. Book value also considers real or actual value of a company and asset as well.
Present value:
This the actual value of any security which will be calculated through different factors and components. For instance, present value measures the future streams of cash flows, payments and worth of the future money in today’s dollars. Present value indicates whether today’s investment gives reasonable return in future. As a result, the present value is widely use in stocks, bonds valuation and financial modelling as well.
Bond:
The term bond defines as it is a long term debt instrument issued by an organization or a government. Company pay fixed rate of return over a particular time-frame on bonds. However, risk on bonds is primarily based on issuer credit worthiness.
Face value:
The face value may be also called par value. The stated value of assets is known as face value. It is basically a financial term which shows value of a security that is stated by the issuer. Furthermore, face value is listed on certificate and shown original cost of a security.
Coupon rate:
The stated rate of return/ yield on a bond is called a coupon rate. The coupon rate is a rate which will pay on fixed income securities in the shape of coupon payment.
Consol:
Consol bond is issued by the government, famously used in Great Britain. A bond that is never matures, infinite period of time. In other words, it is perpetuity in the form of bond.
Perpetual bond:
A perpetual bond is a bond which has no period of time. In other words, earning on this bond continue forever. The valuation of perpetual bond is calculated with the help of this formula.
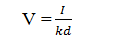
Where:
V = value of bond
i = yield rate
kd = stated rate
When in one period there is more than one compounding’s. Therefore, this formula will be use.
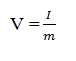
Where:
m = number of compounding
Non zero coupon bond:
The non-zero coupon bond calculate through this formula.

Where:
V = value of bond
i = yield rate
Kd = stated rate
N = number of period
MV = Maturity value
In addition, when in one period there is more than one compounding’s. This formula will be use.
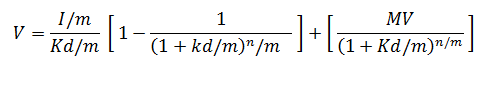
Where:
m = number of compounding
Zero coupon bond:
The zero coupon bond which have stated rate is “0”. To calculating value of zero coupon bonds this formula should be use.

Where:
V = value of bond
MV = maturity value
i = yield rate
When in one period there is more than one compounding’s. Than this formula will be use.
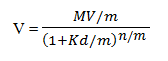
Where:
m = number of compounding
Preferred stock:
Preferred stocks have normally these four characteristics.
- There is fixed rate if dividend will be given on preferred stock
- This is mainly based on the decision of board of directors and, also given preference at the time of dividend.
- The preferred stocks are basically hybrid form of bond, and stock.
- Claim on assets also possible through preferred stocks.
The value of preferred stock calculated through this formula.
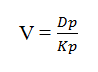
Where:
V = value of bond
Dp = Dividend of preferred stock
Kd = stated rated of preferred stocks.
For example if dividend on preferred stock is $15 and stated rate is 14%. Than what is the actual value of preferred stock?
Common stock valuation:
The holder of common stock is considering actual owner of company. They enjoy the ownership and also bear more risk. As we know there is rule of thumb “more risk and more profit”. The holder of common stock bears more risk, As a result there is more probability that he should earn more profit comparatively with preferred stock. For the valuation of common stock we use dividend discount model. The dividend discounts models are mainly have three types.
Constant growth:
If dividend on common stock is 5%, and this consistently continues. Than valuation of this, we use constant growth model.
V =
Where:
ke = return on equity
D1 = period end dividend
Do = Period beginning dividend
D1 = Do = (1 + g)
i.e. 2 (1+0.05) = $2.10
Zero growth:
In zero growth dividend given continuously but there is no growth. Because firm can’t grow that would be a reason for using zero growth models.
V =
Where:
D1 = period end dividend
ke = return on equity
Phase growth model:
In phase growth model there are different phases of dividend growth. Sometimes dividend growth is constant, sometimes growth is higher and sometime lower. For this reason we called them phase growth model.
Phase growth model real time examples:
- Let’s we assume that a dividend grow in first two periods with the rate of 10%, than next two periods 8%. After four period dividend growth will constant to 4%.
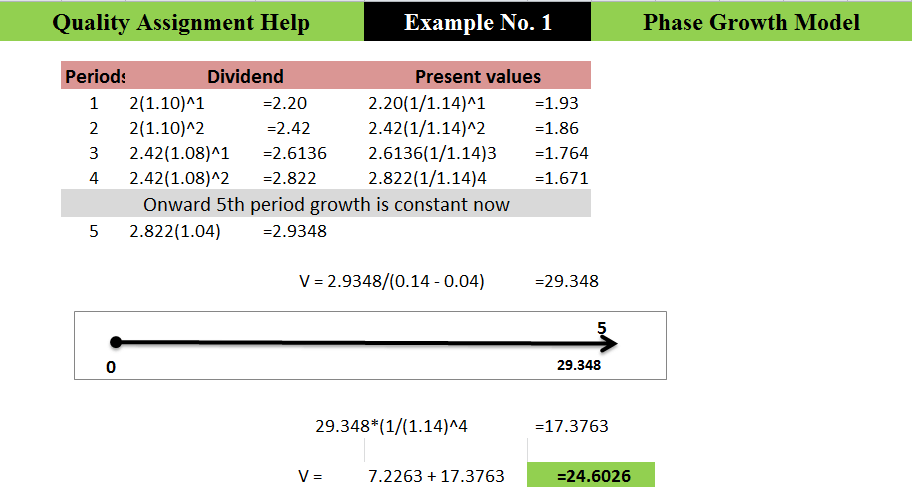
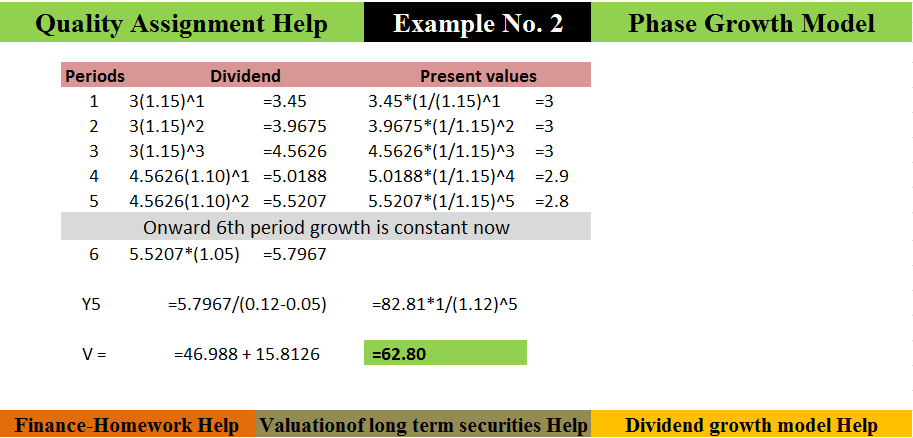
- Let’s now, we assume that a dividend grow in first three periods with the rate of 15%, than next two periods 10%. After fifth period dividend growth will constant to 5%.
Question No. 1:
It is suppose that you are purchasing two different kinds and nature of bonds: one is Alpha, and the second is Beta bonds with following condition:
Alpha:
Face value (pat value) = $1,000
Coupon rate = 10%
Years to maturity (YTM) = 5
Yield’s to maturity = 10%
The current price of Alpha, Pa =?
Beta:
The face value (par value) = $1,000
Coupon rate: 10%
Years to maturity = 3
Yield’s to maturity = 10%
The current price of beta bond, Pb =?
- What are prices of alpha and beta bond?
- Now assume that the beginning of the second year the yield rate in the financial market increase to 20%. After these changes what will be the new worth of each bond? Additionally, what would be the prices of these bonds if the current yield rate was same at 10%? (Note: Now, the years to maturity for Alpha are 4 years, and for the Beta is 2 years.)
- What is your consideration from part b?
- Unlock free of cost solution of this question on quality assignment help sample page click here
Question No. 2:
- Calculate the rate of return of each bond between the first year (t), and the second year (1 +t) when yield rate increase to 20%
- What is the rate of capital gain/loss of each bond?
- If the yield rate declines, Which would you rather be holding, the long term bond (Alpha) or short term bond (Beta)? Why? Which type of the bond has the greatest yield rate risk?
- Unlock free of cost solution of this question on quality assignment help sample page click here
If you still have any confusion regarding any financial help, please don’t hesitate to contact us. Quality assignment help experts are ready to help you. Click here
















Comments are closed.